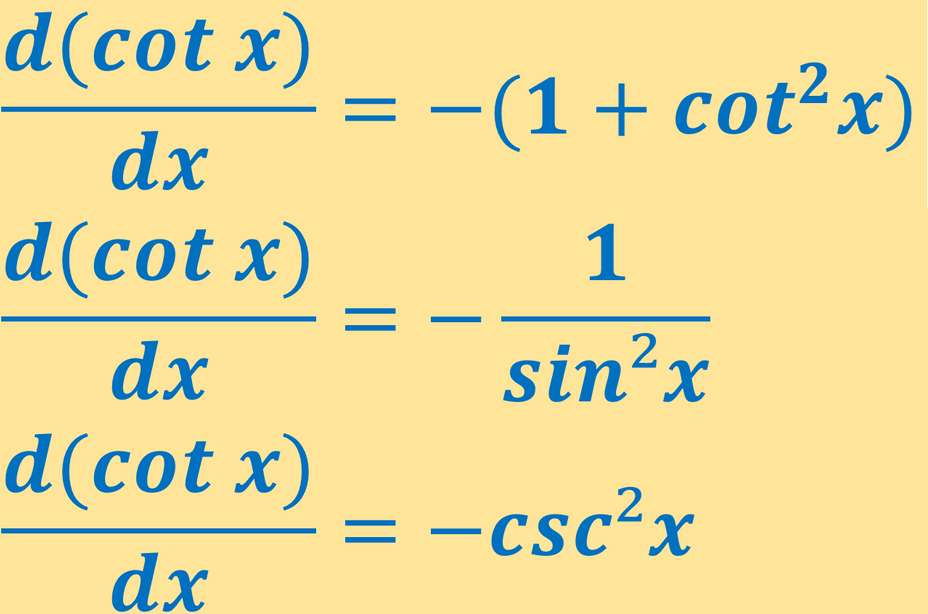Cot x'in türevi -(1+cot² x)'tir.
Cot x'in Türevi Nedir ? Cot x'in türevi -(1+cot² x)'tir.
( co t x ) ′ = − ( 1 + co t 2 x )
d x d ( co t x ) = − ( 1 + co t 2 x )
Cot x'in Türevinin İspatı 1. Yol f ′ ( x ) = h → 0 lim h f ( x + h ) − f ( x ) ( co t x ) ′ = h → 0 lim h co t ( x + h ) − co t x co t ( p + q ) = co t p + co t q co t p . co t q − 1 ( co t x ) ′ = h → 0 lim h co t x + co t h co t x . co t h − 1 − co t x ( co t x ) ′ = h → 0 lim h co t x + co t h co t x . co t h − 1 − co t x . ( co t x + co t h ) ( co t x ) ′ = h → 0 lim h co t x + co t h co t x . co t h − 1 − co t 2 x − co t x . co t h ( co t x ) ′ = h → 0 lim h co t x + co t h − ( 1 + co t 2 x ) ( co t x ) ′ = h → 0 lim [ h 1 . co t x + co t h − ( 1 + co t 2 x ) ] ( co t x ) ′ = h → 0 lim h . ( co t x + co t h ) − ( 1 + co t 2 x ) ( co t x ) ′ = h → 0 lim h . co t x + h . co t h − ( 1 + co t 2 x ) ( co t x ) ′ = h → 0 lim ( h . co t x + h . co t h ) − ( 1 + co t 2 x ) ( co t x ) ′ = h → 0 lim ( h . co t x ) + h → 0 lim ( h . co t h ) − ( 1 + co t 2 x ) ( co t x ) ′ = co t x . h → 0 lim h + h → 0 lim ( h . co t h ) − ( 1 + co t 2 x )
t → 0 l i m ( t . co t t ) = 1 ( co t x ) ′ = co t x .0 + 1 − ( 1 + co t 2 x )
( co t x ) ′ = 0 + 1 − ( 1 + co t 2 x )
( co t x ) ′ = 1 − ( 1 + co t 2 x )
( co t x ) ′ = − ( 1 + co t 2 x )
2. Yol f ′ ( x ) = h → 0 lim h f ( x + h ) − f ( x ) ( co t x ) ′ = h → 0 lim h co t ( x + h ) − co t x co t x = s in x cos x
( co t x ) ′ = h → 0 lim h s in ( x + h ) cos ( x + h ) − s in x cos x
( co t x ) ′ = h → 0 lim h s in x . s in ( x + h ) s in x . cos ( x + h ) − cos x . s in ( x + h )
s in p . cos q − cos p . s in q = s in ( p − q )
( co t x ) ′ = h → 0 lim h s in x . s in ( x + h ) s in [ x − ( x + h )]
( co t x ) ′ = h → 0 lim h s in x . s in ( x + h ) s in ( x − x − h )
( co t x ) ′ = h → 0 lim h s in x . s in ( x + h ) s in ( − h )
s in ( − x ) = − s in x
( co t x ) ′ = h → 0 lim h s in x . s in ( x + h ) − s in h
( co t x ) ′ = h → 0 lim [ h 1 . s in x . s in ( x + h ) − s in h ]
( co t x ) ′ = h → 0 lim h . s in x . s in ( x + h ) − s in h
( co t x ) ′ = h → 0 lim [ h − s in h . s in x . s in ( x + h ) 1 ]
( co t x ) ′ = h → 0 lim h − s in h . h → 0 lim s in x . s in ( x + h ) 1
( co t x ) ′ = − h → 0 lim h s in h . h → 0 lim s in x . s in ( x + h ) 1
t → 0 l i m t s in t = 1
( co t x ) ′ = − 1. s in x . s in ( x + 0 ) 1
( co t x ) ′ = − s in x . s in ( x + 0 ) 1
( co t x ) ′ = − s in x . s in x 1
( co t x ) ′ = − s i n 2 x 1
3. Yol co t x = s in x cos x
( co t x ) ′ = ( s in x cos x ) ′
( v u ) ′ = v 2 u ′ . v − v ′ . u
( co t x ) ′ = s i n 2 x ( cos x ) ′ . sin x − ( s in x ) ′ . cos x
( s in x ) ′ = cos x ( cos x ) ′ = − s in x
( co t x ) ′ = s i n 2 x − s in x . s in x − cos x . cos x
( co t x ) ′ = s i n 2 x − s i n 2 x − co s 2 x
( co t x ) ′ = s i n 2 x − ( s i n 2 x + co s 2 x )
( co t x ) ′ = − s i n 2 x s i n 2 x + co s 2 x
s i n 2 x + co s 2 x = 1
( co t x ) ′ = − s i n 2 x 1
− s i n 2 x 1 = − ( s in x 1 ) 2
( co t x ) ′ = − ( s in x 1 ) 2
s in x 1 = csc x
( co t x ) ′ = − cs c 2 x
( co t x ) ′ = − ( 1 + co t 2 x ) = − s i n 2 x 1 = − cs c 2 x